Table of Contents

• Prequiz...
• Developing the Equations
• 1: Review of diffusion equations
• 2: The two-compartment model
• 3: What does continuous mean?
• 4: Examples of permeability
• 5: A formula for permeability
• 6: The area of the membrane
• 7: The final equations
• Examples
• 8: Rate of flux
• Discrete vs. Continuous
• 9: A metaphor
• 10: Continuous curves
• 11: Discrete curves
• 12: Iterating the discrete curve
• Review
• 13: Summary

 

 

In the Module on Diffusion we looked at diffusion of a substance through air or fluid. In biological systems the membrane provides a barrier around the cell so we need to look at diffusion through cell membranes.

Learning Outcomes

After completing this module you should be able to:

• Apply the diffusion equations to movement of a substance across a membrane which is selectively permeable.

Equation deja vu

Remember back in the diffusion module, we used an equation to relate the rate of diffusion (rate of flow of particles through an area, i.e. flux) to the steepness of the concentration gradient? Well, in case you've forgotten, that equation was:

Continuous form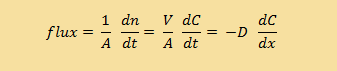

 

and we also discussed how this "continuous" equation can be made into a "discrete" equation:

 

Discrete form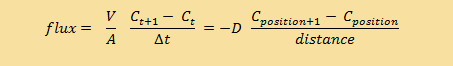

In these equations, n is the number of particles, t is the time, C is the concentration, V is the volume, A is the area through which the particles are diffusing, D is the diffusion coefficient and dC/dx  (or ΔC/Δx ) is the gradient.

It is important to understand that BOTH of these equations refer to the same basic phenomenon -- particles moving at random, with the net result that particles move from areas of high concentration to areas of low concentration. BOTH of these equations describe what's happening in the same way -- the concentration gradient is defined by the difference in concentrations per distance between them. Remember that the units of flux are mol m^-2 s^-1.

So why are we using two different equations for the same thing??? Sometimes, a discrete equation is an "easier" version of a continuous equation, and sometimes it's the other way around, the continuous is an "easier" version of the discrete. In this case, the discrete is easier, but the continuous is more realistic. The discrete is an approximation or simplification of reality. The discrete equation tells us a lot about the process without needing to get involved in the truly tricky kind of mathematics required to work with the continuous version (which require partial differential equations -- look them up if you want to know more!).

Clipart for this module thanks to Arthur's Clipart, FreeImages.co.uk, Clipart Heaven